Question

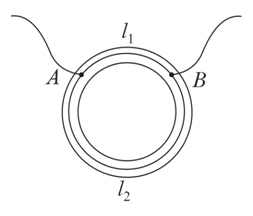
A ring is made of a wire having a resistance $R_{0}=12\Omega$ . Find the points $A$ and $B$ as shown in the figure at which a current carrying conductor should be connected so that the resistance $R$ of the sub circuit between these points is equal to $\frac{8}{3}\Omega$ :

• A. $\frac{\ell _{1}}{\ell _{2}}=\frac{3}{8}$
• B. $\frac{\ell _{1}}{\ell _{2}}=\frac{1}{2}$
• C. $\frac{\ell _{1}}{\ell _{2}}=\frac{5}{8}$
• D. $\frac{\ell _{1}}{\ell _{2}}=\frac{1}{3}$

Answer 1

Answer: (B)

Initially $R_{1}+R_{2}=12\Omega$
Across point A & B, both branches will be in parallel connection.
$\frac{R_{1} R_{2}}{R_{1} + R_{2}}=\frac{8}{3}\Omega$
$\Rightarrow R_{1}R_{2}=32$
Now $R_{2}-R_{1}=\sqrt{\left(R_{1} + R_{2}\right)^{2} - 4 R_{1} R_{2}}$
$\Rightarrow \sqrt{12^{2} - 4 \times 32}=4\Omega$
So $R_{1}=4\Omega$ and $R_{2}=8\Omega$
For a uniform cross-sectional area, resistance will be proportional to the length of the wire.
Therefore, $\frac{\ell _{1}}{\ell _{2}}=\frac{4}{8}=\frac{1}{2}$