Question

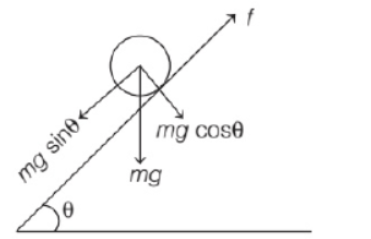
A rigid body of mass $M$ and radius $R$ rolls without slipping on an inclined plane of inclination $\theta$, under gravity. Match the type of body Column I with magnitude of the force of friction Column II.

Column I		Column II
A	For ring	I	$\frac{ Mg \sin \theta}{2.5}$
B	For solid sphere	II	$\frac{ Mg \sin \theta}{3}$
C	For solid cylinder	III	$\frac{ Mg \sin \theta}{3.5}$
D	For hallow cylinder	IV	$\frac{ Mg \sin \theta}{2}$

• A. A=IV, B=III ,C= II,D=IV
• B. A=I, B=II ,C=IV ,D= IV
• C. A=II, B=I ,C= IV,D= III
• D. A=II, B=IV ,C=I ,D= III

Answer 1

Answer: (A)

Given, mass, radius of rigid body be $m$ and $R$ respectively,
$g$ is acceleration due to gravity and $\theta$ is inclination.
Let $I$ be the moment of inertia, then
$I=n m R^2$
Taking force along inclined
$m g \sin \theta-f=m a \ldots \text { (i) }$

where, $f$ is friction force and $a$ is acceleration.
$\tau=I \alpha=f R \ldots \text { (ii) }$
where, $\tau=$ torque,
$I=$ moment of inertia
and $\alpha=$ angular acceleration $=\frac{a}{R}$.
Substituting in Eq. (ii), we get
$\Rightarrow m g \sin \theta-n m a=m a $
$\Rightarrow g \sin \theta=a(n+1) $
$ \Rightarrow a=\frac{g \sin \theta}{n+1}$
Substituting in Eq. (iii), we get
$f =n m a$
$ =n m \frac{g \sin \theta}{n+1} \ldots$
(A) For ring, $I=m R^2$
$\therefore n=1$
$\text { and } f_{\text {ring }}=\frac{m g \sin \theta}{1+1}=\frac{m g \sin \theta}{2}$
(B) For solid sphere, $I=\frac{2}{5} m R^2$
$ \therefore n=\frac{2}{5} $
$f_{\text {solid sphere }}=\frac{\frac{2}{5} m g \sin \theta}{\frac{2}{5}+1} $
$=\frac{2 m g \sin \theta}{7} $
$ =\frac{m g \sin \theta}{3.5} $
$ \therefore n=\frac{2}{5}$
$f_{\text {solid sphere }}=\frac{\frac{2}{5} m g \sin \theta}{\frac{2}{5}+1}$
$=\frac{m g \sin \theta}{3}$
$ \therefore n=\frac{1}{2}$
$ f_{\text {solid cylinder }}=\frac{\frac{1}{2} m g \sin \theta}{\frac{1}{2}+1} $ $=\frac{m g \sin \theta}{3}$
(D) For hollow cylinder $I=M R^2$
$\therefore n=1$
$f_{\text {hollow cylinder }}=\frac{m g \sin \theta}{1+1}$
$=\frac{m g \sin \theta}{2}$
$\therefore$ Hence, $A \rightarrow 4, B \rightarrow 3, C \rightarrow 2, D \rightarrow 4$ is the correct.