Q.
A ray of light falls on a transparent sphere with centre $C$ as shown in the figure. The ray emerges from sphere parallel to line $A B .$ The refractive index of sphere is
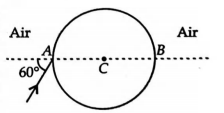
Ray Optics and Optical Instruments
Solution:
