Q.
A projectile is thrown with velocity of $50 \,m / s$ towards an inclined plane from ground such that it strikes the inclined plane perpendicularly. The angle of projection of the projectile is $53^{\circ}$ with the horizontal and the inclined plane is inclined at an angle of $45^{\circ}$ to the horizontal. Find the distance (in $m$ ) between the point of projection and the foot of inclined plane.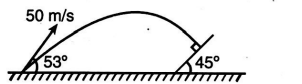
Motion in a Plane
Solution:
