Q.
A potentiometer wire $A B$ having length $L$ and resistance $12 r$ is joined to a cell $D$ of emf $\varepsilon$ and internal resistance $r$. A cell $C$ having emf $\frac{\varepsilon}{2}$ and internal resistance $3 r$ is connected. The length AJ at which galvanometer as shown in figure shows no deflection is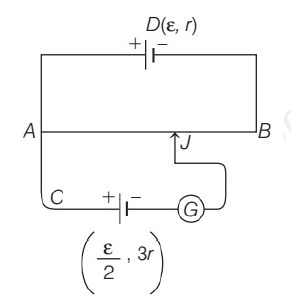
MHT CETMHT CET 2021
Solution: