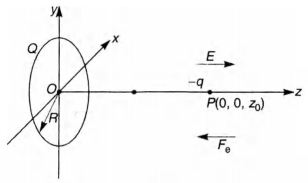
Q. A positively charged thin metal ring of radius $R$ is fixed in the x-y plane with its centre at the origin $O$. A negatively charged particle $P$ is released from rest at the point $(0,0,z_0) $ where $z_0>0. $ Then the motion of P is
IIT JEEIIT JEE 1998Electrostatic Potential and Capacitance
Solution:
Let Q be the charge on the ring, the negative charge - q is
released from point P (0,0, $z_0). $ The electric field at P due to
the charged ring will be along positive z-axis and its
magnitude will be
$\, \, \, \, \, E= \frac {1}{4 \pi \varepsilon_0} \frac {Qz_0}{(R^2+z_0^2)^{3/2}} $
E = 0 at eentre of the ring because $z_0=0 $
Force on charge at P will be towards centre as shown, and its
magnitude is
$\, \, \, \, \, F_e=qE= \frac {1}{4 \pi \varepsilon_0}. \frac {Qq}{(R^2+z_0^2)^{3/2}}.z_0 \, \, \, \, \, \, \, \, ...(i) $
Similarly, when it crosses the origin, the force is again
towards centre O.
Thus, the motion of the particle is periodic for all values of $z_0 $
lying between 0 and $\infty $
Secondly, if $z_0 < < R, (R^2+ z_0^2)^{3/2}=R^3 $
$\, \, \, \, \, F_e= \frac {1}{4 \pi \varepsilon_0}. \frac {Qq}{R^3}.z_0 [From \, Eq.(i)] $
i.e. the restoring force $F_e \propto -z_0. $ Hence, the motion of the
particle will be simple harmonic. (Here negative sign implies
that the force is towards its mean position.)