Q.
A positive charge $+Q$ is fixed at a point A. Another positively charged particle of mass $m$ and charge $+ q$ is projected from a point $B$ with velocity $u$ as shown in the figure. The point $B$ is at the large distance from $A$ and at distance $d$ from the line $A C$. The initial velocity is parallel to the line $A C$. The point $C$ is at very large distance from $A$. The minimum distance (in meter) of $+ q$ from $+ Q$ during the motion is $d(1+\sqrt{A})$. Find the value of $A$.
[Take $Oq =4 \pi \varepsilon_0 mu ^2 d$ and $d =(\sqrt{2}-1)$ meter.]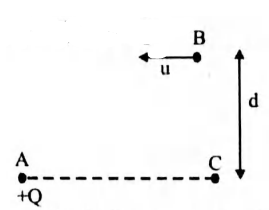
Electric Charges and Fields
Solution:
