Q.
A particle of mass $M$ and charge $q$ is at rest at the midpoint between two other fixed similar charges each of magnitude $Q$ placed a distance $2d$ apart. The system is collinear as shown in the figure. The particle is now displaced by a small amount $x (x < < d)$ along the line joining the two charges and is left to itself. It will now oscillate about the mean position with a time period ($\epsilon_0$ = permittivity of free space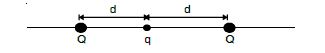
Solution: