Question

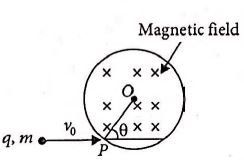
A particle of charge $q$ and mass $m$ is projected with a velocity $v_{0}$ towards a circular region having uniform Magnetic field $B$ perpendicular and into the plane of paper, from point $P$ as shown in figure. $R$ is the radius and $O$ is the centre of the circular region. If the line $OP$ makes an angle $\theta$ with the direction of $v_{0}$ then the value of $v_{0}$ so that particle passes through $O$ is

• A. $\frac{qBR}{m\,sin\, \theta}$
• B. $\frac{qBR}{2m\,sin\, \theta}$
• C. $\frac{2qBR}{m\,sin\, \theta}$
• D. $\frac{3qBR}{2m\,sin\, \theta}$

Answer 1

Answer: (B)

Let $r$ be the radius of circular path. If the particle passes through $O$, then from the given figure,

$sin\, \theta = \frac{R/2}{r}=\frac{R}{2r} \dots (i)$
As $r=\frac{mv_{0}}{qB}$
$\therefore v_{0}=\frac{qBr}{m}$
$=\frac{qBR}{2m\,sin\,\theta}$ (Using (i))