Question

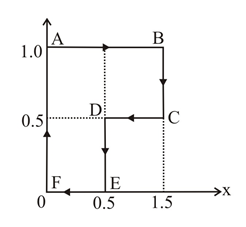
A particle is moved along a path $ABCDEFA,$ as shown in figure, in presence of a force $\vec{F}=\left(\alpha y \hat{i} + 2 \alpha x \hat{ȷ}\right)N$ , Where $x$ and $y$ are in meter and $\alpha =-1\,Nm^{- 1}$ . The work done on the particle by this force $\vec{F}$ $\left(\text{in} J\right)$ is $\frac{p}{100}$ , find $p$ .

Answer 1

As $\alpha =-1$
$\therefore \vec{ F}=-\underset{1}{\underbrace{y \hat{i} - x \hat{j}}}-x\hat{j}$
This is now a perfect differential format whose work done is zero for a complete cycle. Hence for $-xj$ only WD needs to be calculated.
$\therefore W=1\times 0.5+0.5\times 0.5$
$=0.5+0.25$
$=0.75\,J$