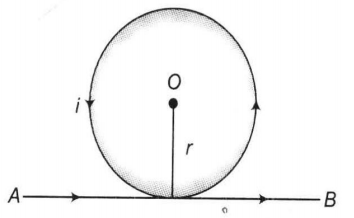
Q.
A part of a long wire carrying a current i is bent into a
circle of radius r as shown in figure. The net magnetic
field at the centre O of the circular loop is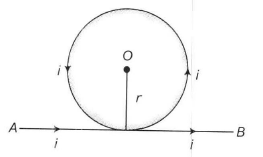
Jharkhand CECEJharkhand CECE 2010
Solution:
The magnitude of the magnetic field at point O due to straight
part of wire is
$ B_1= \frac {\mu _0i}{2 \pi r} $
$B_1 $ is perpendicular to the plane of the page, directed upwards
(right hand palm rule I).
The field at the centre O due to the current loop of radius r is
$ B_2= \frac {\mu _0i}{2r} $
$B_2 $ is also perpendicular to the page, directed upward (right
hand screw rule).
$\therefore $ Resultant field at O is
$B_1+B_2= \frac {\mu _0i}{2r}\bigg (\frac {1}{\pi }+1 \bigg ) $
$ = \frac {\mu_0i}{2 \pi r}( \pi+1) $