Q.
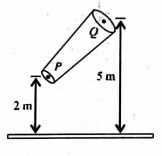
A non-viscous liquid of constant density $1000\, kg\, m ^{-3}$ flows in a streamline motion along a tube of variable cross-section. The tube is kept inclined in the vertical plane as shown in figure. The areas of cross-section of the tube at P and Q at heights of 2 m and 5 m are respectively $4 \times 10^{-3} \,m ^{2}$ and $8 \times 10^{-3} \,m ^{2}$. The velocity of liquid at point is $1 \,m \,s ^{-1}$. The velocity of liquid at $Q$ is
Mechanical Properties of Fluids
Solution: