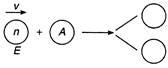
Q. A neutron moving with a velocity $v$, collides elastically with an atom of mass number $A$. The collision is head on. If the initial kinetic energy of a neutron is $e$, then the kinetic energy of neutron after the collision is :
J & K CETJ & K CET 2001
Solution:
In elastic collision, kinetic energy and momentum both are conserved.
Let $v_{1}$ be velocity after collision
$v_{1}=\left(\frac{m_{1}-m_{2}}{m_{1}+m_{2}}\right) u_{1}+\left(\frac{2 m_{2}}{m_{1}+m_{2}}\right) u_{2}$
Given, $u_{1}=v, u_{2}=0, m_{1}=1, m_{2}=A $
$\therefore v_{1}=\left(\frac{1-A}{1+A}\right) v$ Initial kinetic energy of neutron $E=\frac{1}{2} m_{1} v_{1}^{2}=\frac{1}{2} \times 1 \times\left(\frac{1-A}{1+A}\right)^{2}$
$\therefore \frac{E}{E}=\left(\frac{1-A}{1+A}\right)^{2} $
$\Rightarrow E=\left(\frac{1-A}{1+A}\right)^{2} E$
Final KE of neutron
$E=\frac{1}{2} m_{1} v_{1}^{2}=\frac{1}{2} \times 1 \times\left(\frac{1-A}{1+A}\right)^{2} $
$\therefore \frac{E}{E}=\left(\frac{1-A}{1+A}\right)^{2}$
$ \Rightarrow E=\left(\frac{1-A}{1+A}\right)^{2} E$