Q.
A monochromatic light is travelling in a medium of refractive index $𝑛 = 1.6$. It enters a stack of glass layers from the bottom side at an angle $\theta=30^{\circ}$. The interfaces of the glass layers are parallel to each other. The refractive indices of different glass layers are monotonically decreasing as $n_{m}=n-m\Delta n,$ where $n_m$ is the refractive index of the $m^{th}$ slab and $\Delta n = 0.1$ (see the figure). The ray is refracted out parallel to the interface between the $(m - 1)^{th}$ and $m^{th}$ slabs from the right side of the stack. What is the value of $m$?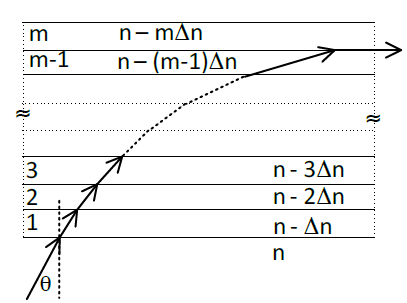
JEE AdvancedJEE Advanced 2017
Solution: