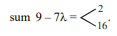1. Tardigrade
  2. Question
  3. Mathematics
  4. A line L passing through the point P (2,4,3) is perpendicular to both the lines (x-1/2)=(y+3/1)=(z-2/4) and (x-2/3)=(y+1/2)=(1-z/2). If the position vector of point Q on L is (a, b, c) such that (P Q)2=357 then a + b + c can be

Question Error Report

Thank you for reporting, we will resolve it shortly

Back to Question

Q. A line $L$ passing through the point $P (2,4,3)$ is perpendicular to both the lines $\frac{x-1}{2}=\frac{y+3}{1}=\frac{z-2}{4}$ and $\frac{x-2}{3}=\frac{y+1}{2}=\frac{1-z}{2}$. If the position vector of point $Q$ on $L$ is $(a, b, c)$ such that $(P Q)^2=357$ then $a + b + c$ can be

Vector Algebra

Solution:

Equation of $L: \frac{x-2}{10}=\frac{y-4}{-16}=\frac{z-3}{-1}=\lambda $
$\overrightarrow{ PQ }=10 \lambda \hat{ i }-16 \lambda \hat{ j }-\lambda \hat{ k } $
$\Rightarrow 35 \lambda^2=357 \Rightarrow \lambda= \pm 1$
$Q=(2+10 \lambda, 4-16 \lambda, 3-\lambda)$

Solution Image