Q.
A $L$ shaped rod of mass $M$ is free to rotate in a vertical plane about axis $AA^{′}$ as shown in figure. Maximum angular acceleration of rod is
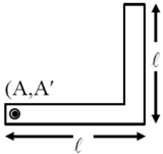
NTA AbhyasNTA Abhyas 2020System of Particles and Rotational Motion
Solution:
