Question

A horizontal stretched string, fixed at two ends, is vibrating in its fifth harmonic according to the equation,
$y(x,t)=(0.01m)[sin(628m^{-1})x]cos[(628 \, s^{-1}) t ]. $
Assuming $\pi =3.14, $ the correct statement(s) is (are)

• A. the number of nodes is 5
• B. the length of the string is 0.25 m
• C. the maximum displacement of the mid-point of the string from its equilibrium position is 0.01 m
• D. the fundamental frequency is 100 Hz

Answer 1

Answer: (B), (C)

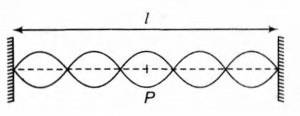
Number of nodes $=6$
From the given equation, we can see that
$
\begin{array}{c}
k=\frac{2 \pi}{\lambda}=62.8 m^{-1} \\
\therefore \lambda=\frac{2 \pi}{62.8} m=0.1 m \\
l=\frac{5 \lambda}{2}=0.25 m
\end{array}
$
The mid-point of the string is $P$, an antinode
$\therefore $ maximum displacement $=0.01 m$
$
\begin{array}{l}
\omega=2 \pi f=628 s^{-1} \\
\therefore f=\frac{628}{2 \pi}=100 Hz
\end{array}
$
But this is fifth harmonic frequency.
$\therefore $ Fundamental frequency $f_{0}=\frac{f}{5}=20 Hz$