Q.
A hollow cylinder has a charge $q$ coulomb within it. If $\phi$ is the electric flux, in unit of $V m$, associated with the curved surface $B$, the flux linked with the plane surface $A$, in unit of $V m$, will be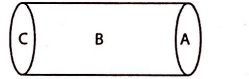
NEETNEET 2022
Solution: