1. Tardigrade
  2. Question
  3. Physics
  4. A hockey player receives a corner shot at a speed of 15 m/s at an angle of 30? with the y-axis and then shoots the ball of mass 100 g along the negative x-axis with a speed of 30 m/s. If it remains in contact with the hockey stick for 0.01 s, the force imparted to the ball in the x-direction is

Question Error Report

Thank you for reporting, we will resolve it shortly

Back to Question

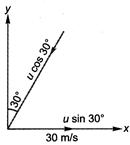
Q. A hockey player receives a corner shot at a speed of 15 m/s at an angle of 30? with the y-axis and then shoots the ball of mass 100 g along the negative x-axis with a speed of 30 m/s. If it remains in contact with the hockey stick for 0.01 s, the force imparted to the ball in the x-direction is

ManipalManipal 2008Laws of Motion

Solution:

If a constant force $ \overset{\to }{\mathop{F}}\, $ is applied on a body for short interval of time $ \Delta t $ , then the impulse this force is $ F\times \Delta t $ .
Since, impulse = change in momentum $ (\Delta p) $
$ \therefore $ $ F\times \Delta t=\Delta p $
$ \Rightarrow $ $ F=\frac{\Delta p}{\Delta t} $ Change in $ x- $ direction $ F=\frac{m[30-(-15\sin {{30}^{o}})]}{0.01} $ $ F=\frac{0.1\times 37.5}{0.01}=375\,\,N $ Note: Unit of impulse is same as that of momentum.

Solution Image