Question

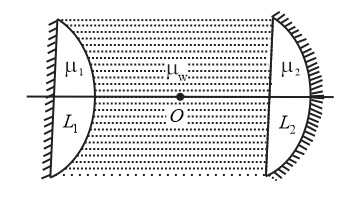
A cylindrical tube filled with water $\left(\left(\mu \right)_{w} = \frac{4}{3}\right)$ is closed at its both ends by two thin, silvered plano-convex lenses, as shown in the figure. Refractive index of lenses $L_{1}$ and $L_{2}$ are $2.0$ and $1.5$ , while their radii of curvatures are $5 \, cm$ and $9 \, cm$ , respectively. A point object is placed somewhere at a point $O$ on the axis of the cylindrical tube. If it is found that all the images formed by multiple refractions and reflections coincide with the object, then the distance between both the lenses is

• A. $8 \, cm$
• B. $18 \, cm$
• C. $10 \, cm$
• D. $14 \, cm$

Answer 1

Answer: (B)

For the lens $L_{1}$ , the ray must move parallel to the axis after refraction, for the image to coincide with the object.
$\frac{\mu _{1}}{\infty}+\frac{\mu _{w}}{x}=\frac{\mu _{1 \, } - \mu _{w}}{R_{1}}$ , where $x$ is the distance of the object from the lens $L_{1}$
$\frac{4/3}{x}=\frac{2-4/3}{5}$
$\Rightarrow x=10cm$
For the lens $L_{2}$ , the ray must appear to come from the centre of curvature after refraction, for the image to coincide with the object.
$\frac{\mu _{2}}{- R_{2}}+\frac{\mu _{w}}{y}=\frac{\mu _{1 \, } - \mu _{w}}{\infty }$ , where $y$ is the distance of the object from the lens $L_{2}$
$y=R_{2}\frac{4/3}{3/2}=8cm$
Distance between the two lenses = $10cm+8cm=18cm$