Q.
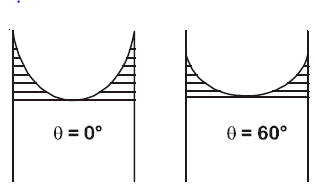
A cylindrical capillary tube of $0.2\, mm$ radius is made by joining two capillaries $T_1$ and $T_2$ of different materials having water contact angles of $0^\circ$ and $60^\circ$, respectively. The capillary tube is dipped vertically in water in two different configurations, case I and II as shown in figure. Which of the following option(s) is(are) correct?
[Surface tension of water = 0.075 N/m, density of water = $1000\, kg/m^3$, take $g = 10 \,m/s^2$]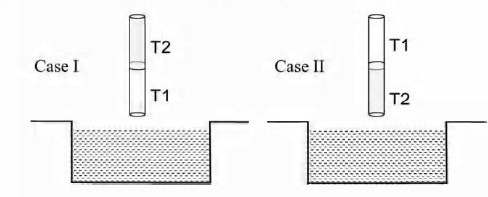
Solution:
For A : $h=\frac{2T\,cos\,60^{\circ}}{\rho gr}=\frac{2\times0.075\times1}{10^{3}\times10\times2\times10^{-4}\times2}\times100\,cm=3.75\,cm$
$\Rightarrow $ Option A is correct
For B : $h=\frac{2T\,cos\,0}{\rho gr}=7.5\,cm$
$\Rightarrow $ Option B is correct
For C : Angle of contact will adjust to make h = 5 cm
For D : The shape of meniscus is different in the two cases
$\Rightarrow $ Correction is different