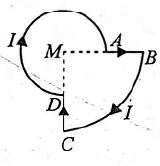
Q.
A current $I$ is flowing through a loop. The direction of the current and the shape of the loop are as shown in the figure The magnetic field at the centre of the loop is $\frac{\mu_{0} I}{R}$ times (Here, $MA=R, MB=2R, \angle DMA= 90^{\circ})$
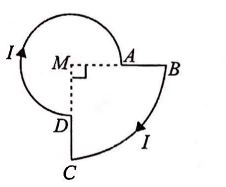
Moving Charges and Magnetism
Solution: