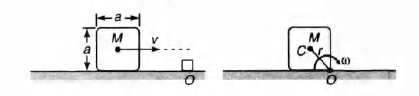
Q.
A cubical block of side a moving with velocity $v$ on a horizontal smooth plane as shown. It hits a ridge at point $O$. The angular speed of the block after it hits $O$ is
IIT JEEIIT JEE 1999System of Particles and Rotational Motion
Solution:
$r^{2}=\frac{a^{2}}{2}$
Net torque about $O$ is zero.
Therefore, angular momentum $(L)$ about $O$ will be conserved, or $L_{i}=L_{f}$
$M v\left(\frac{a}{2}\right)=I_{0} \omega=\left(I_{c m}+M r^{2}\right) \omega$
$=\left\{\left(\frac{M a^{2}}{6}\right)+M\left(\frac{a^{2}}{2}\right)\right\} \omega$
$=\frac{2}{3} M a^{2} \omega$
$\omega=\frac{3 v}{4 a}$