Question

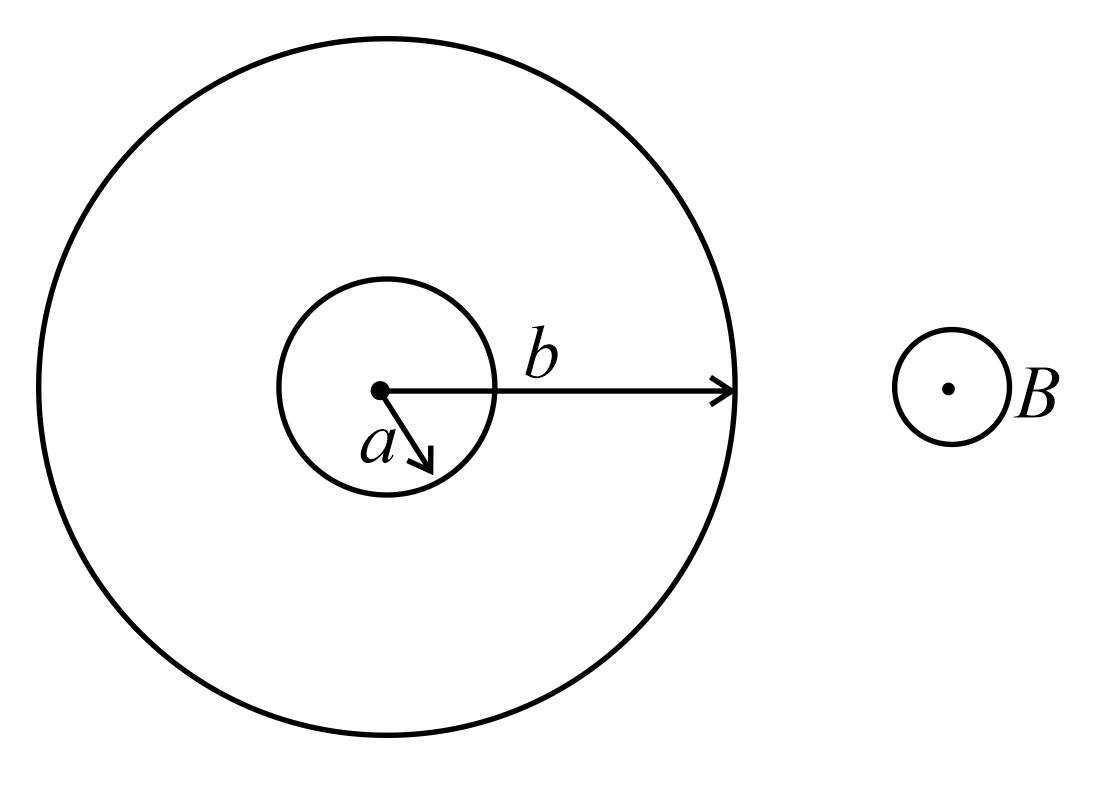
A conducting ring of circular cross-section with inner and outer radii $a$ and $b$ is made out of a material of resistivity $\rho $ . The thickness of the ring is $h$ . It is placed coaxially in a vertical cylindrical region of magnetic field, $B=krt$ , where $k$ is a positive constant, $r$ is the distance from the axis and $t$ is the time. If the current through the ring is, $I=\left(\frac{k h}{\alpha p}\right)\left[b^{3} - a^{3}\right]$ , then what is the value of $\alpha $ ?

Answer 1

The magnetic flux through the area of radius $r$ is,
$\phi=\displaystyle \int _{0}^{r}krt2\pi rdr=\frac{2 \pi k t r^{3}}{3}$ .
Emf induced is the rate of change of the magnetic flux,
$e=\left|\frac{d \phi}{d t}\right|=\frac{2 \pi k r^{3}}{3}$ .
The net induced current in terms of emf and resistance,
$I=\displaystyle \int _{a}^{b}\frac{2 \pi r^{3} k h d r}{3 \left(\right. 2 \pi r \left.\right)}=\frac{k h}{9 \rho }\left(b^{3} - a^{3}\right)$ .