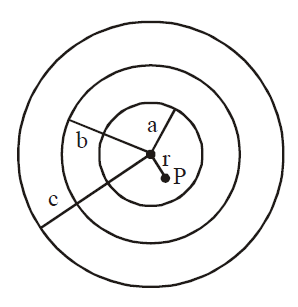
Question

A charge $Q$ is distributed over three concentric spherical shells of radii $a, b, c (a < b < c )$ such that their surface charge densities are equal to one another. The total potential at a point at distance r from their common centre, where $r < a$, would be :

• A. $\frac{Q}{4\pi\varepsilon_{0} \left(a+b+c\right)} $
• B. $\frac{Q \left(a+b+c\right)}{4\pi\varepsilon_{0} \left(a^{2} +b^{2} +c^{2}\right)} $
• C. $\frac{Q}{12\pi\varepsilon_{0}} \frac{ab+bc+ca}{abc} $
• D. $\frac{Q}{4\pi\varepsilon_{0}} \frac{\left(a^{2} + b^{2} +c^{2}\right)}{\left(a^{3} +b^{3} +c^{3}\right)} $

Answer 1

Answer: (B)

Potential at point P, $V = \frac{kQ_{a}}{a} + \frac{kQ_{b}}{b} + \frac{kQ_{c}}{c} $
$ \because Q_{a} : Q_{b} : Q_{c} : : a^{2} :b^{2} :c^{2} $
$ \left\{\text{since} \sigma_{a} = \sigma_{b} =\sigma_{c}\right\}$
$ \therefore Q_{a} = \left[\frac{a^{2}}{a^{2}+b^{2}+c^{2}}\right]Q $
$Q_{b} = \left[\frac{b^{2}}{a^{2}+ b^{2} +c^{2}}\right]Q $
$ Q_{c} = \left[\frac{c^{2}}{a^{2}+b^{2} +c^{2}}\right]Q $
$ V = \frac{Q}{4 \pi\in_{0}} \left[\frac{\left(a+b+c\right)}{a^{2}+b^{2}+c^{2}}\right] $