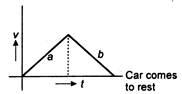
Q. A car starts from rest, moves with an acceleration $a$ and then decelerates at a constant rate $b$ for some time to come to rest. If the total time taken is $t$. The maximum velocity of car is given by:
BHUBHU 2005
Solution:
From equation of motion, we have
$ v=u+at $
Since, body starts from rest
$ u=0 $ .
Let $ {{t}_{1}} $ be time when body accelerates and $ {{t}_{2}} $
when it decelerates. $ \therefore $ $ t={{t}_{1}}+{{t}_{2}} $
$ \Rightarrow $ $ {{t}_{2}}=t-{{t}_{1}} $
$ \therefore $ $ v=0+a{{t}_{1}} $ ...(1)
When, car finally comes to rest
$ v=0 $
$ \therefore $ $ 0=v-b(t-{{t}_{1}}) $ ...(2)
From Eqs. (1) and (2), we get
$ {{t}_{1}}=\frac{b}{a+b}t,v=\frac{ab}{a+b}t $