Q.
A capacitor is made of a flat plate of area $A$ and a second plate of stair-like structure as shown in the figure. The area of each stair is $\frac{A}{3}$ and the height is $d$. The capacitance of the arrangement is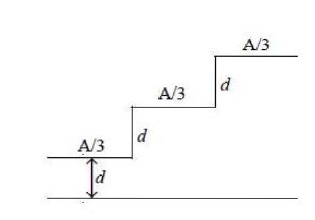
AP EAMCETAP EAMCET 2019
Solution:
