Question

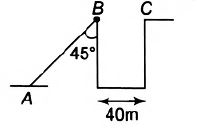
A body is projected up a smooth inclined plane with velocity V from the point A as shown in the figure. The angle of inclination is $45^{o}$ and the top is connected to a well of diameter $40$ m. If the body just manages to cross the well, what is the value of $V$? Length of inclined plane is $20\sqrt{2}\,m$.

• A. $40\,\,ms^{-1}$
• B. $40\sqrt{1} \,\,ms^{-1}$
• C. $20\,\,ms^{-1}$
• D. $20\sqrt{1} \,\,ms^{-1}$

Answer 1

Answer: (D)

Angle of projection from B is $45^{o}$. As the body is able to cross the well of diameter $40$ m, hence
or $R=\frac{v^{2}}{g} \,,or\,\,v=\sqrt{gR}$
$v=\sqrt{10\times40} -20\,\,ms^{-1}$
On the inclined plane, the retardation is:
g sin $\alpha=g$ sin $45^{o}=\frac{10}{\sqrt{2}}ms^{-2}$
Using $v^{2} - u^2 = 2ax$
$(20)^{2} -u^{2}=2\times(-\frac{10}{\sqrt{2}})\times 20\sqrt{2}$
$u=20\sqrt{2} \,\,ms^{-1}\,,\,$
i.e., $v=20\sqrt{2}\,\,ms^{1}$