Question

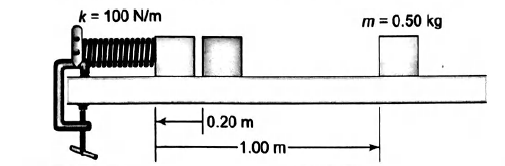
A block with mass $0.50\, kg$ is forced against a horizontal spring of negligible mass, compressing the spring a distance of $0.20\, m$ (fig). When released, the block moves on a horizontal table top for $1.00\, m$ before coming to rest. The spring constant k is $100\, N/m$. What is the coefficient of kinetic friction, $\mu_{k},$ between the block and the table?

• A. 0.40
• B. 0.50
• C. 0.25
• D. None of these

Answer 1

Answer: (A)

The initial and final kinetic energies are both zero, so the work done by the spring is the negative of the work done by friction,
or $\frac{1}{2}kx^{2}=\mu_{k} mgl,$ where $l$ is the distance the block moves. Solving for$\mu_{k},$
$\mu_{k}=\frac{(1 / 2) k x^{2}}{m g l}$
$=\frac{(1 / 2)(100\, N / m )(0.20\, m )^{2}}{(0.50\, kg )\left(10\, m / s ^{2}\right)(1.00\, m )}$
$=0.40$