Q.
A block of mass $m$, lying on a smooth horizontal surface, is attached to a spring (of negligible mass) of spring constant $k$. The other end of the spring is fixed, as shown in the figure. The block is initally at rest in its equilibrium position. If now the block is pulled with a constant force $F$, the maximum speed of the block is :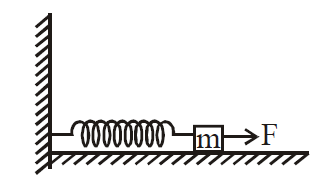
Solution: