Q.
A block of mass $m$ is sliding down an inclined plane with constant speed.At a certain instant $t_{0}$, its height above the ground is $h$. The coefficient of kinetic friction between the block and the plane is $\mu$. If the block reaches the ground at a later instant $t_{g}$, then the energy dissipated by friction in the time interval $\left(t_{g}-t_{0}\right)$ is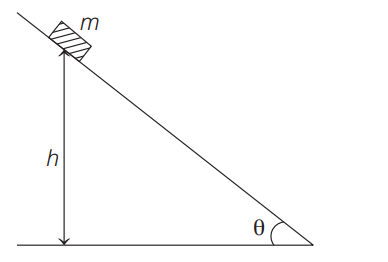
KVPYKVPY 2012
Solution: