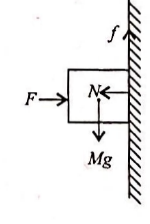
Question

A block of mass $M$ is held against a rough vertical wall by pressing it with a finger. If the coefficient of friction between the block and the wall is $\mu$ and the acceleration due to gravity is $g$, what is the minimum force required to be applied by the finger to hold the block against the wall?

• A. $\mu$ $Mg$
• B. $Mg$
• C. $\frac{Mg}{\mu}$
• D. $2 \mu$ $Mg$

Answer 1

Answer: (C)

Let $F$ be the force applied by the finger on the block. $N$ is normal reaction of the wall on the block. $Mg$ is weight of block acting vertically downwards. $f$ is the force of friction between the wall and the block which acts upwards as shown in the figure.
The block will not fall, if $f = Mg$
$\mu N=Mg$ $\quad\left(\because\,f=\mu N\right)$
$\mu F=Mg$ $\left(\because\,N=F\right)$ $=F=\frac{M\,g}{\mu}$