Q.
A block $M$ hangs vertically at the bottom end of a uniform rope of constant mass per unit length. The top end of the rope is attached to a fixed rigid support at $O$. A transverse wave pulse (Pulse 1) of wavelength $\lambda_{0}$ is produced at point $O$ on the rope. The pulse takes time $T_{OA}$ to reach point $A$. If the wave pulse of wavelength $\lambda_{0}$ is produced at point $A$ (Pulse 2) without disturbing the position of $M$ it takes time $T_{AO}$ to reach point $O$. Which of the following options is/are correct?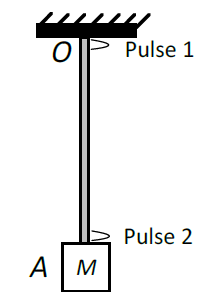
Solution:
