1. Tardigrade
  2. Question
  3. Physics
  4. A block is moving on an inclined plane making an angle 45° with the horizontal and the coefficient of friction is μ The force required to just push it up the inclined plane is 3 times the force required to just prevent it from sliding down. If we define N=10 μ, then N is

Question Error Report

Thank you for reporting, we will resolve it shortly

Back to Question

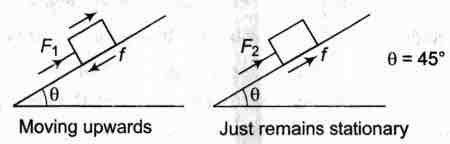
Q. A block is moving on an inclined plane making an angle $45^\circ$ with the horizontal and the coefficient of friction is $\mu$ The force required to just push it up the inclined plane is $3$ times the force required to just prevent it from sliding down. If we define $N=10\,\mu, $then $N$ is

IIT JEEIIT JEE 2011Laws of Motion

Solution:

$F_1 - mg \sin\theta + \mu mg \cos\theta$
$F_2=mg \sin \theta- \mu mg \cos \theta$
Given that $F_1=3F_2$ or $(sin 45^{\circ} +\mu \cos 45^{\circ})$
$= 3 (sin 45^{\circ} - \mu \cos 45^{\circ})$
On solving, we get$=0.5 \therefore N=10\,\mu =5$

Solution Image