Q.
A block $C$ of mass m is moving with velocity $ {{v}_{o}} $ and collides elastically with block $A$ of mass m and connected to another block $B$ of mass $2m$ through spring of spring constant $k$. What is $k$, if $ x_o $ is compression of spring when velocity of $A$ and $B$ is same?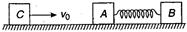
Delhi UMET/DPMTDelhi UMET/DPMT 2006Work, Energy and Power
Solution: