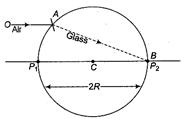
Q. A beam of parallel light rays is incident on a solid transparent sphere of refractive index $ \mu $ . If a point image is produced just at the back of the sphere, what is the refractive index of the sphere?
AMUAMU 2014Ray Optics and Optical Instruments
Solution:
Let $C$ be the centre and $P_{1}$ and $P_{2}$ the poles of the sphere.
$A$ ray $OA$parallel to a diameter is first refracted into the glass along $AB$.
For refraction at $A$ from air to glass, we have
$\frac{\mu}{v}-\frac{1}{u}-\frac{\mu-1}{R}$
Here $u =\infty$
and $v=2 R$
$\therefore \frac{\mu}{2 R}-\frac{1}{\infty}=\frac{\mu-1}{R}$
$\frac{\mu}{2 R}=\frac{\mu-1}{R}$
$\mu=2 \mu-2$
$\mu=2$