Q.
A bead of mass $m$ can slide on a frictionless wire as shown in figure. Because of the given shape of the wire, near $P$, the bottom point, it can be approximated as parabola. $\operatorname{Near} P$, the potential energy of the bead is given $U=c x^{2}$ where $c$ is a constant and $x$ is measured from $P$. The bead, if displaced slightly from point $P$ will oscillate about $P$. The period of oscillation is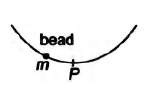
Oscillations
Solution: