Q.
A battery with internal resistance of $4 \,\Omega$ is connected to a circuit consisting three resistances, $R, \,2 R$ and $4R$ (see following figure). If the power generated in the circuit is highest, then the magnitude of $R$ must be
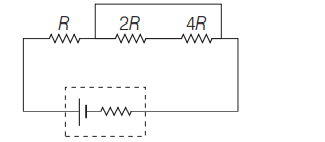
TS EAMCET 2018
Solution: