Q.
A ball is thrown from ground at an angle $\theta $ with horizontal and with an initial speed $u_{0}.$ For the resulting projectile motion, the magnitude of average velocity of the ball up to the point when it hits the ground for the first time is $V_{1}.$ After hitting the ground, the ball rebounds at the same angle $\theta $ but with a reduced speed of $u_{0}/\alpha .$ Its motion continues for a long time as shown in figure. If the magnitude of average velocity of the ball for entire duration of motion is $0.8V_{1},.$ the value of $\alpha $ is
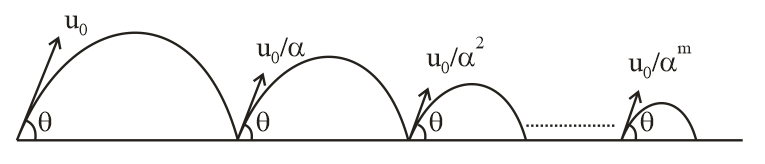
NTA AbhyasNTA Abhyas 2022
Solution:
 $V_{\text{avg }}=\frac{R_{1} + R_{2} + R_{3} + \ldots \ldots }{T_{1} + T_{2} + T_{3} + \ldots \ldots }$
$V_{\text{avg }}=\frac{R_{1} + R_{2} + R_{3} + \ldots \ldots }{T_{1} + T_{2} + T_{3} + \ldots \ldots }$