1. Tardigrade
  2. Question
  3. Physics
  4. A and B are two sources generating sound waves. A listener is situated at C. The frequency of the source at A is 500 Hz. A, now, moves towards C with a speed 4 m/s. The number of beats heard at C is 6. When A moves away from C with speed 4 m/s, the number of beats heard at C is 18. The speed of sound is 340 m/s. The frequency of the source at B is :

Question Error Report

Thank you for reporting, we will resolve it shortly

Back to Question

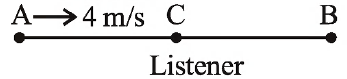
Q. $A$ and $B$ are two sources generating sound waves. A listener is situated at $C$. The frequency of the source at $A$ is $500\, Hz$. A, now, moves towards $C$ with a speed $4\, m/s$. The number of beats heard at $C$ is $6$. When $A$ moves away from $C$ with speed $4 \,m/s$, the number of beats heard at $C$ is $18$. The speed of sound is $340\, m/s$. The frequency of the source at $B$ is :Physics Question Image

JEE MainJEE Main 2013Waves

Solution:

$f = 500\, Hz$
Case 1 : When source is moving towards stationary listener
apparent frequency $\eta'=\eta\left(\frac{v}{v-v_{s}}\right)$
$=500\left(\frac{340}{336}\right)=506\,Hz$
Case 2 : When source is moving away from the stationary listener
$\eta''=\eta\left(\frac{v}{v+v_{s}}\right)=500\left(\frac{340}{344}\right)=494\,Hz$
In case 1 number of beats heard is 6 and in case 2 number ofbeats heard is 18 therefore frequency of the source at $B = 512\, Hz$

Solution Image