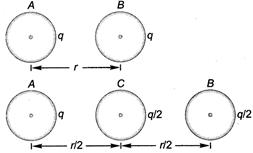
Q. A and B are two identical spherical charged bodies which repel each other with force F, kept at a finite distance. A third uncharged sphere of the same size is brought in contact with sphere B and removed. It is then kept at mid-point of A and B. Find the magnitude of force on C.
ManipalManipal 2008Electric Charges and Fields
Solution:
From Coulombs law, the force of attraction between two charged particles $ (q) $ , kept at distance r apart is
when two identical spheres are brought in contact, charge on them is equalized, hence total charge on $ C $ is equally shared when brought in contact with sphere $ B $ having a charge $ q $ . Therefore, charge on $ B $ and $ C $ is $ \frac{q}{2} $ .
From Coulombs law, the force on $ C $ is $ {{F}_{C}}=\frac{q\times q/2}{4\pi {{\varepsilon }_{0}}{{(r/2)}^{2}}}-\frac{(q/2)(q/2)}{4\pi {{\varepsilon }_{0}}{{(r/2)}^{2}}} $
$=\frac{qq}{4\pi {{\varepsilon }_{0}}{{r}^{2}}}(2-1)=F $
Note: The force will be opposite because $ A $ and $ B $ spheres will repel the third sphere.