Q. $\frac{1}{12}ML^{2}$ is the moment of inertia of a rod about an axis through its centre and perpendicular to it. The rod is bent from the middle at an angle $60^\circ $ . Find the moment of inertia of the bent rod about the same axis. ( $M$ is the mass and $L$ is the length of the rod)
NTA AbhyasNTA Abhyas 2020
Solution:
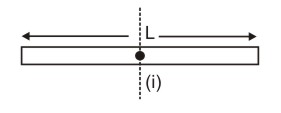
Given $I_{1}=\frac{1}{12}ML^{2}$
now as per the question
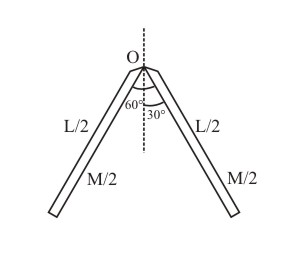
Both half rodes are in same plane.
now
$I=\frac{2 ml^{2}}{3}sin^{2}\theta $
where $m$ is mass of each rod, $l$ is its length and $\theta $ is half of angle between them.
So
$I=\frac{2 \left(\frac{M}{2}\right) \left(\frac{L}{2}\right)^{2}}{3}\times \left(sin\right)^{2}30^\circ $
$I=\frac{ML^{2}}{48}$

Given $I_{1}=\frac{1}{12}ML^{2}$
now as per the question

Both half rodes are in same plane.
now
$I=\frac{2 ml^{2}}{3}sin^{2}\theta $
where $m$ is mass of each rod, $l$ is its length and $\theta $ is half of angle between them.
So
$I=\frac{2 \left(\frac{M}{2}\right) \left(\frac{L}{2}\right)^{2}}{3}\times \left(sin\right)^{2}30^\circ $
$I=\frac{ML^{2}}{48}$