- Tardigrade
- Question
- Physics
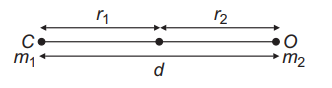
- The key feature of Bohr's theory of spectrum of hydrogen atom is the quantisation of angular momentum when an electron is revolving around a proton. We will extend this to a general rotational motion to find quantized rotational energy of a diatomic molecule assuming it to be rigid. The rule to be applied is Bohr's quantization condition. It is found that the excitation frequency from ground to the first excited state of rotation for the C O molecule is close to (4/π) × 1011 Hz. - In a C O molecule, the distance between C ( mass =12 amu ) and O( mass =16 amu ), where 1 amu =(5/3) × 10-27 kg, is close to
Q.
The key feature of Bohr's theory of spectrum of hydrogen atom is the quantisation of angular momentum when an electron is revolving around a proton. We will extend this to a general rotational motion to find quantized rotational energy of a diatomic molecule assuming it to be rigid. The rule to be applied is Bohr's quantization condition.
It is found that the excitation frequency from ground to the first excited state of rotation for the molecule is close to .
- In a molecule, the distance between and , where , is close to
Solution:
and
Now,